Diagonals of operators

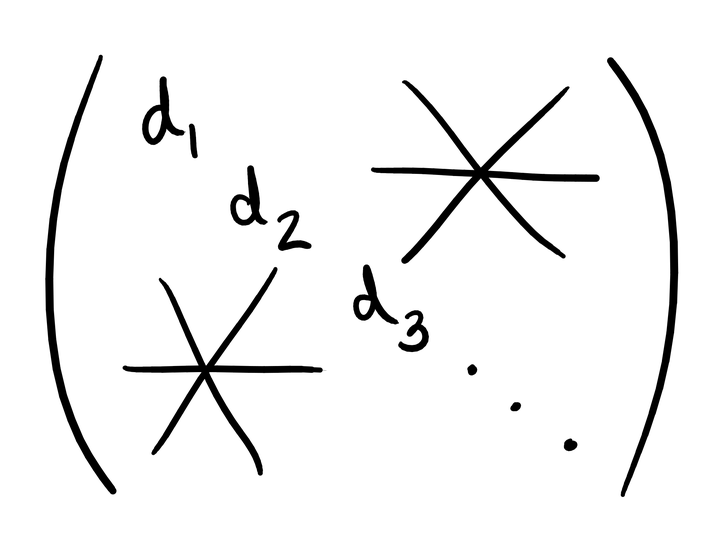
What is a diagonal?

By a diagonal of a bounded operator $T \in B(\Hil)$ acting on a separable Hilbert space we mean a sequence $(\angles{Te_n,e_n})_{n=1}^{\infty}$ where $\set{e_n}_{n=1}^{\infty}$ is an orthonormal basis of $\Hil$. The orthonormal basis is not fixed, and so $T$ has many diagonals. We will use $\D(T)$ to denote the set of all diagonals of $T$.

A useful equivalent viewpoint is to fix the orthonormal basis and consider the diagonals of the operators $UTU^{-1} = UTU^{*}$ in the unitary orbit $\uorbit(T)$ relative to this fixed orthonormal basis. If $E$ denotes the canonical trace-preserving conditional expectation onto the subalgebra of diagonal operators determined by this fixed basis (i.e., $E$ denotes the operation of “taking the main diagonal”), then there is a natural identification between $\D(T)$ and $E(\uorbit(T))$ via the *-isomorphism $\diag : \ell^{\infty} \to E(B(\Hil))$. As such, sometimes we regard elements of $E(\uorbit(T)) \subseteq B(\Hil)$ as diagonals of $T$ even though they are operators as opposed to sequences.

History

There is by this point a long history of studying the diagonals of operators. One of the early results in this area is the so-called Schur–Horn theorem which characterizes the diagonals of a selfadjoint $n \times n$ matrix as the collection of real sequences which are majorized by the eigenvalue sequence, repeated according to multiplicity.

There has been a program spanning over two decades now whose ultimate goal is to characterize the diagonals of all selfadjoint operators on a separable infinite dimensional Hilbert space.