Numerical range

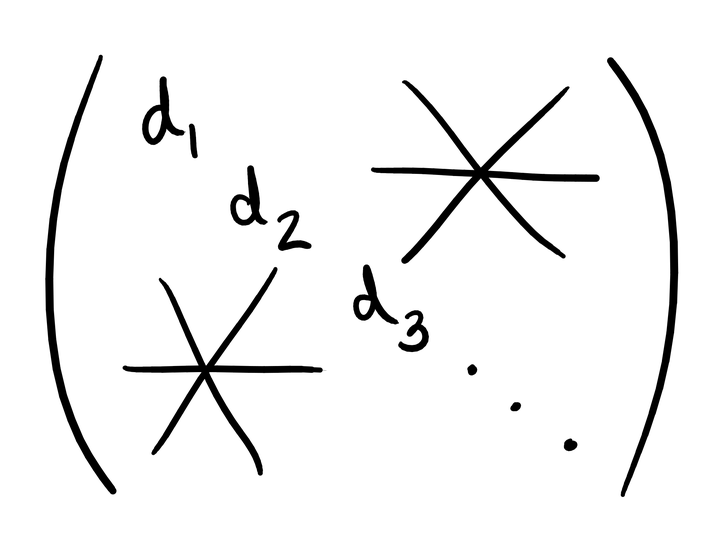
For $A \in B(H)$, the numerical range $W(A)$ is the image of the unit sphere of $H$ under the continuous quadratic form $x \mapsto \langle Ax,x \rangle$, where $\langle \cdot,\dot \rangle$ denotes the inner product on $H$. Of course, the numerical range has a long history but perhaps the most impactful result is the Toeplitz–Hausdorff Theorem which asserts that the numerical range is convex [Toe-1918-MZ], [Hau-1919-MZ]; an intuitive proof is given by Davis in [Dav-1971-CMB]. In this paper we are interested in unitarily invariant generalizations of the numerical range and their associated properties, especially convexity and its relation to majorization.

By considering an alternative definition of the numerical range, some generalizations become readily apparent. Notice that $$ W(A) = { \langle Ax,x \rangle \mid x \in H, \Vert x \Vert = 1 } = { \operatorname{Tr}(PA) \mid P\ \text{is a rank-1 projection} }. $$ As Halmos recognized in [[Hal-1964-ASM]], one could generalize this by fixing $k \in \N$ and requiring $P$ to be a rank-$k$ projection. In this way, we arrive at the $k$-numerical range

$$W_k(A) := \left( \operatorname{Tr}\Big(\frac{1}{k}PA\Big) \vert P\ \text{is a rank-$k$ projection} \right).$$

The normalization constant $\frac{1}{k}$ is actually quite natural; among other things, it ensures $W_k(A)$ is bounded independent of $k$ by $\Vert A \Vert$. In [section 12, Ber-1963][[Ber-1963]], Berger proved a few fundamental facts about the $k$-numerical range including its convexity, as well as the inclusion property $W_{k+1}(A) \subseteq W_k(A)$. We will see shortly that these convexity and inclusion properties are actually consequences of more general phenomena.

In [[FW-1971-GMJ]], Fillmore and Williams examined $W_k(A)$, but restricted their attention to the finite dimensional setting. There they established $$ W_k(A) = \left{ \frac{1}{k} \operatorname{Tr}(XA) \middle\vert 0 \le X \le I, \operatorname{Tr} X = k \right}, $$

which was generalized by Goldberg and Straus to the $C$-numerical range, as we describe below. Moreover, Fillmore and Williams showed that if $A \in M_n(\mathbb{C})$ is normal, then $$ W_k(A) = \operatorname{conv} \left{ \frac{1}{k} \sum_{i=1}^k \lambda_i \middle\vert \text{$\lambda_i$ is an eigenvalue of $A$, repeated at most according to multiplicity} \right}, $$ which says that the extreme points of $W_k(A)$ are contained in the set of averages of $k$-eigenvalues of $A$ (allowing repetitions according to multiplicity). This is a clear analogue of the standard fact for numerical ranges that $W(A) = \operatorname{conv} \sigma(A)$ when $A \in M_n(\mathbb{C})$ is normal.

In order to further generalize the $k$-numerical range, yet another new perspective is necessary. The unitary group $\mathcal{U}$ of $B(H)$ acts by conjugation on $B(H)$, and the orbit $\mathcal{U}(C)$ of an operator $C \in B(H)$ under this action is called the unitary orbit. When $P$ is any rank-$k$ projection ($k < \infty$), $\mathcal{U}(P)$ consists of all rank-$k$ projections in $B(H)$. Therefore, if $P$ is a rank-$k$ projection, then $$ W_k(A) = \left{ \operatorname{Tr}(XA) \middle\vert X \in \mathcal{U}\left(\frac{1}{k}P\right) \right}. $$

The above representation of the $k$-numerical range suggests the natural generalization to the $C$-numerical range, $$ W_C(A) := { \operatorname{Tr}(XA) \mid X \in \mathcal{U}(C) }. $$ Of course, this requires $\operatorname{Tr}(XA)$ to make sense, which can be achieved in several different ways, each investigated by various authors. In [[Wes-1975-LMA]], Westwick considered $W_C(A)$ when $C$ is a finite rank selfadjoint operator and proved that $W_C(A)$ is convex by means of Morse theory. When $\operatorname{dim} H = n < \infty$ so that $B(H) \cong M_n(\mathbb{C})$, $W_C(A)$ is well-defined for an arbitrary $C \in M_n(\mathbb{C})$. The $C$-numerical range was first studied in this generality by Goldberg and Straus in [GS-1977-LAA]. There, they proved a generalization of \eqref{eq:knr-majorization-description} for an arbitrary selfadjoint matrix $C$, which we extend to the infinite dimensional setting. Chi-Kwong Li provides in [[Li-1994-LMA]] a comprehensive survey of the properties of the $C$-numerical range in finite dimensions, including the highlights which we now describe. When $C$ is selfadjoint the $C$-numerical range is convex, but this may fail even if $C$ is normal [[Wes-1975-LMA]], [AT-1983-LMA]. However, the $C$-numerical range is always star-shaped relative to the star center $\operatorname{Tr}(C) \big(\frac{1}{n} \operatorname{Tr}(A)\big)$ [[CT-1996-LMA]]. Moreover, there is a set $P_C(A)$ associated to the pair $C,A$ called the $C$-spectrum of $A$ which, when $C$ is a rank-1 projection, coincides with the usual spectrum of $A$; Then when $A$ is normal and $C$ is selfadjoint, $W_C(A) = \operatorname{conv} P_C(A)$ Theorem 4, Mar-1979-ANYAS, which generalizes \eqref{eq:knr-extreme-points}.

In the recent paper [DvE-2020-LaMA], Dirr and vom Ende study a generalization of the $C$-numerical range to the infinite dimensional setting. In this case, it again becomes necessary to ensure that the trace $\operatorname{Tr}(XA)$ is well-defined, which they naturally enforce by requiring $C$ to be trace-class. In [DvE-2020-LaMA], they prove extensions of some finite dimensional results by means of limiting arguments. As a result of these limiting arguments, all of their major results pertain to the \emph{closure} $\overline{W_C(A)}$ of the $C$-numerical range. Dirr and vom Ende prove that $\overline{W_C(A)}$ is star-shaped and that any element of $\operatorname{Tr}(C) W_{ess}(A)$ is a star center Theorem 3.10, DvE-2020-LaMA. They asked explicitly Open Problem (b), DvE-2020-LaMA whether $W_C(A)$ is convex without taking the closure, and we provide a partial answer. Moreover, they show that $\overline{W_C(A)}$ is convex whenever $C$ is selfadjoint or $A$ is a rotation and translation of a selfadjoint operator Theorem 3.8, DvE-2020-LaMA. Additionally, they prove that if $C,A$ are both normal, $A$ is compact and the eigenvalues of either $C$ or $A$ are collinear, then $\overline{W_C(A)} = \operatorname{conv}(\overline{P_C(A)})$ Corollary 3.1, DvE-2020-LaMA.

In this paper we introduce and investigate a natural modification of the $C$-numerical range with $C$ trace-class which we call the orbit-closed $C$-numerical range, denoted $W_{\mathcal{O}(C)}(A)$. The only difference between $W_{\mathcal{O}(C)}(A)$ and $W_C(A)$ is that the former allows $X$ which are \emph{approximately} unitarily equivalent (in trace norm) to $C$, that is, $$ W_{\mathcal{O}(C)}(A) := { \operatorname{Tr}(XA) \mid X \in \mathcal{O}(C) }, $$ where $\mathcal{O}(C) := \overline{\mathcal{U}(C)}^{\norm{\cdot}_1}$. Considering closures of unitary orbits in various operator topologies serves an important purpose and has precedent in the literature, especially in relation to majorization.

This relatively small difference between $W_C(A)$ and $W_{\mathcal{O}(C)}(A)$ has significant consequences. In particular, for $C$ selfadjoint we give a characterization of $W_{\mathcal{O}(C)}(A)$ in terms of majorization which is an appropriate extension to infinite dimensions of [Theorem 1.2, FW-1971-GMJ][[FW-1971-GMJ]] (included herein as \eqref{eq:knr-majorization-description}) and its generalization Theorem 7, GS-1977-LAA, and whose proof is inspired by [Theorem 2.14, DS-2017-PEMSIS][[DS-2017-PEMSIS]]. Because in general $W_C(A) \not= W_{\mathcal{O}(C)}(A)$, necessarily $W_C(A)$ cannot enjoy this same characterization. Moreover, this majorization characterization of $W_{\mathcal{O}(C)}(A)$ is the backbone of this paper and it provides a gateway to the rest of our major results. One immediate corollary is the convexity of $W_{\mathcal{O}(C)}(A)$ when $C$ is selfadjoint which generalizes and provides an independent and purely operator-theoretic proof of Westwick’s theorem [[Wes-1975-LMA]] for $C$ a finite rank selfadjoint operator. Another proof of Westwick’s theorem which utilized the majorization approach is provided by Poon. [[Poo-1980-LMA]] using a result of Goldberg and Straus Theorem 7, GS-1977-LAA. However, because of implicit dependence on Birkhoff’s theorem [Bir-1946-UNTRA] the specific techniques seem not to generalize to infinite rank $C$.

In addition, $W_{\mathcal{O}(C)}(A)$ is a \emph{conservative} modification of $W_C(A)$ in the sense that $W_C(A) \subseteq W_{\mathcal{O}(C)}(A) \subseteq \overline{W_C(A)}$, and moreover, if $C$ is finite rank, then $W_{\mathcal{O}(C)}(A) = W_C(A)$. Therefore, the orbit-closed $C$-numerical range constitutes an alternate natural extension of the $C$-numerical range to the infinite dimensional (and infinite rank) setting. Moreover, because $\overline{W_{\mathcal{O}(C)}(A)} = \overline{W_C(A)}$, all of Dirr and vom Ende’s results (which concern the closure of the $C$-numerical range) are inherited by the orbit-closed $C$-numerical range.

Our main results are summarized in the list below. Here $\lambda(C)$ denotes the eigenvalue sequence of a compact operator $C$, $\prec$, $\prec\prec$ denote majorization and submajorization, and $\sigma_{\mathcal{O}(C)}(A)$ denotes the $\mathcal{O}(C)$-spectrum.

1. $W_{\mathcal{O}(C)}(A) = W_C(A)$ if $C$ is finite rank.
2. $\overline{W_{\mathcal{O}(C)}(A)} = \overline{W_C(A)}$.
3. The map $(C,A) \mapsto W_{\mathcal{O}(C)}(A)$ is continuous.
4. If $C \in \mathcal{L_1}^{sa}$, then $$ W_{\mathcal{O}(C)}(A) = { \operatorname{Tr}(XA) \mid X \in \mathcal{L}_1^{sa}, \lambda(X) \prec \lambda(C) }. $$
5. If $C \in \mathcal{L_1}^{sa}$, then $W_{\mathcal{O}(C)}(A)$ is convex.
6. If $C,C' \in \mathcal{L_1}^{sa}$ and $\lambda(C) \prec \lambda(C')$, then $W_{\mathcal{O}(C)}(A) \subseteq W_{\mathcal{O}(C')}(A)$.
7. If $C \in \mathcal{L_1}^{sa}$, $A \in \mathcal{K}$, then $$ \overline{W_{\mathcal{O}(C)}(A)} = { \operatorname{Tr}(XA) \mid X \in \mathcal{L_1}^{sa}, \lambda(X) \prec\prec \lambda(C)} = W_{\mathcal{O}(C \oplus \mathbf{0})}(A \oplus \mathbf{0}) $$ as long as $\mathbf{0}$ acts on a space of dimension at least $\operatorname{rank} C$.
8. For $C \in \mathcal{L_1}^+$, $W_{\mathcal{O}(C)}(A)$ is closed if for every $\theta$, $\operatorname{rank} (\Re(e^{i\theta}A)-m_{\theta}I)_+ \ge \operatorname{rank} C$, where $m_{\theta} := \max \sigma_{ess}(\Re(e^{i\theta} A))$.
9. If $C \in \mathcal{L_1}^+$, then $$ W_{\mathcal{O}(C)}(A_1 \oplus A_2) = \operatorname{conv} \quad \bigcup_{\mathclap{\quad C_1 \oplus C_2 \in \mathcal{O}(C)}} \ \big( W_{\mathcal{O}(C_1)}(A_1) + W_{\mathcal{O}(C_2)}(A_2) \big). $$
10. If $C \in \mathcal{L_1}^+$, $A \in \mathcal{K}$ normal, then $W_{\mathcal{O}(C)}(A) = \operatorname{conv} \sigma_{\mathcal{O}(C)}(A)$.
11. If $C \in \mathcal{L}_1^+$ with $\operatorname{dim} \ker C \in {0,\infty}$, and $A \in B(\Hil)$ is diagonalizable, then $W_C(A)$ is convex.